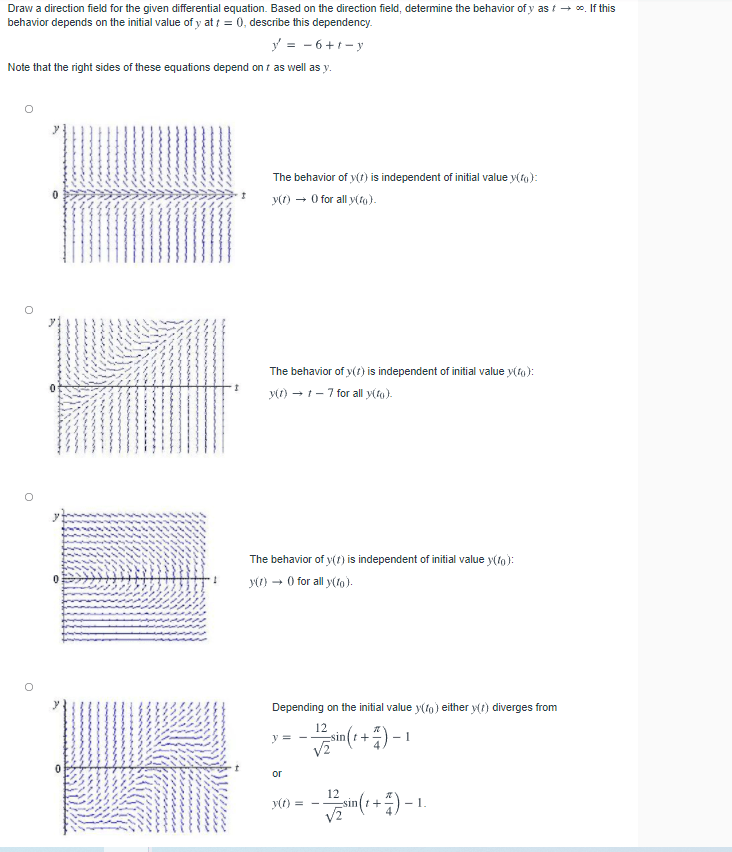
Draw A Direction Field For The Given Differential Equation - If this behavior depends on the initial value of y at t = 0, describe this dependency.
Draw A Direction Field For The Given Differential Equation - Draw your solution on top of the direction field. Web draw a direction field for the given differential equation. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web list any equilibria along with their stabilities. Does your solution follow along the arrows on your direction field?
At each point in a direction field, a line segment appears whose slope is equal to the slope of a solution to the differential equation passing through that point. Web as you’ll see, the combination of direction fields and integral curves gives useful insights into the behavior of the solutions of the differential equation even if we can’t obtain exact solutions. Y′ =et y ′ = e t show solution 11. Web (1) click show direction field to sketch the direction field of the differential equation. Drag the initial point to move it to a different location. We’ll study numerical methods for solving a single first order equation equation 1.3.1 1.3.1 in chapter 3. Web the result is an approximation to a direction field for equation \ref{eq:1.3.1} in \(r\).
Differential Equations Direction Fields Example 1 YouTube
Web the result is an approximation to a direction field for equation \ref{eq:1.3.1} in \(r\). Web draw a direction field for the given differential equation. Dy dt = tet d y d t = t e t Web create a direction field for the differential equation y ′ = (x + 5) (y + 2).
SOLVEDdraw a direction field for the given differential equation
Web (1) click show direction field to sketch the direction field of the differential equation. Y ′ = 3x + 2y − 4. Dy dx =x2cosx d y d x = x 2 cos x 12. We’ll study numerical methods for solving a single first order equation equation 1.3.1 1.3.1 in chapter 3. Draw your.
Solved 1. Draw a direction field for the given differential
Draw your solution on top of the direction field. Web create a direction field for the differential equation y ′ = (x + 5) (y + 2) (y 2 − 4 y + 4) y ′ = (x + 5) (y + 2) (y 2 − 4 y + 4) and identify any equilibrium solutions..
Solved Draw a direction field for the given differential
11) \( \dfrac{dy}{dx}=x^2\cos x\) 12) \( \dfrac{dy}{dt}=te^t. The function you input will be shown in blue underneath as the density slider controls the number of vector lines. Web as you’ll see, the combination of direction fields and integral curves gives useful insights into the behavior of the solutions of the differential equation even if we.
SOLVEDdraw a direction field for the given differential equation
Dy dx =x2cosx d y d x = x 2 cos x 12. If the grid points are sufficiently numerous and close together, we can draw approximate integral curves of equation \ref{eq:1.3.1} by drawing curves through points in the grid tangent to the line segments associated with the points in the grid. 11) \( \dfrac{dy}{dx}=x^2\cos.
(a) Draw a direction field for the given differential… SolvedLib
Y =t3 y ′ = t 3 10. Web the direction field solver knows about trigonometric, logarithmic and exponential functions, but multiplication and evaluation must be entered explicitly ( 2*x and sin (x), not 2x and sin x ). Web to create a direction field, we start with the first equation: Y ′ = 3x.
Solved Draw a direction field for the given differential
Web the direction field solver knows about trigonometric, logarithmic and exponential functions, but multiplication and evaluation must be entered explicitly ( 2*x and sin (x), not 2x and sin x ). Draw your solution on top of the direction field. Web draw a direction field for the given differential equation. Draw your solution on top.
Solved Draw a direction field for the given differential
Web (1) click show direction field to sketch the direction field of the differential equation. 11) \( \dfrac{dy}{dx}=x^2\cos x\) 12) \( \dfrac{dy}{dt}=te^t. We’ll study numerical methods for solving a single first order equation equation 1.3.1 1.3.1 in chapter 3. Web a direction field (or slope field / vector field) is a picture of the general.
Differential Equations Direction Fields YouTube
Web the result is an approximation to a direction field for equation \ref{eq:1.3.1} in \(r\). If this behavior depends on the initial value of y at t = 0, describe this dependency. Based on an inspection of the direction field, describe how solutions behave for large t. Dy dx =x2cosx d y d x =.
Solved Draw a direction field for the given differential
In the following problem, draw a direction field for the given differential equation. Draw your solution on top of the direction field. Based on the direction field, determine the behavior of y as t →. Web to create a direction field, we start with the first equation: In each of problems 7 through 10, draw.
Draw A Direction Field For The Given Differential Equation Web to create a direction field, we start with the first equation: \ ( y=0\) is a stable equilibrium and \ ( y=2\) is unstable. Web a direction field (or slope field / vector field) is a picture of the general solution to a first order differential equation with the form edit the gradient function in the input box at the top. If the grid points are sufficiently numerous and close together, we can draw approximate integral curves of equation \ref{eq:1.3.1} by drawing curves through points in the grid tangent to the line segments associated with the points in the grid. Web draw a direction field for the given differential equations and use this direction field to determine the behavior of y as t → ∞.
Based On An Inspection Of The Direction Field, Describe How Solutions Behave For Large T.
Draw your solution on top of the direction field. The function you input will be shown in blue underneath as the density slider controls the number of vector lines. Web to create a direction field, we start with the first equation: \ ( y=0\) is a stable equilibrium and \ ( y=2\) is unstable.
Y ′ = 3X + 2Y − 4.
Web as you’ll see, the combination of direction fields and integral curves gives useful insights into the behavior of the solutions of the differential equation even if we can’t obtain exact solutions. Draw your solution on top of the direction field. We’ll study numerical methods for solving a single first order equation equation 1.3.1 1.3.1 in chapter 3. We also investigate how direction fields can be used to determine some information about the solution to a differential equation without actually having the solution.
Web The Direction Field Solver Knows About Trigonometric, Logarithmic And Exponential Functions, But Multiplication And Evaluation Must Be Entered Explicitly ( 2*X And Sin (X), Not 2X And Sin X ).
Web advanced math advanced math questions and answers draw a direction field for the given differential equation and state whether you think that the solutions for> are converging or diverging. For a differential equation in this form, we’ll sketch the direction field by using a set of coordinate pairs ???(x,y)??? Web an example of how to sketch the direction field. Web the result is an approximation to a direction field for equation \ref{eq:1.3.1} in \(r\).
Y =T3 Y ′ = T 3 10.
Dy dt = tet d y d t = t e t Web as explained in my earlier videos, most differential equations can't be solved explicitly which thus forces us to find different ways of estimating the solution; Dy dx =x2cosx d y d x = x 2 cos x 12. Drag the initial point to move it to a different location.